Mortality improvements and CMI_2019: frequently asked questions (FAQs)

1. The latest version of the CMI Model, CMI_2019

What happened to mortality in 2019?

Standardised mortality rates in England & Wales were 3.8% lower in 2019 than in 2018. This is the largest fall in mortality rates since 2011.

Mortality in 2019 was the lowest that we have seen in England & Wales.

How has the CMI Model changed compared to CMI_2018?

CMI_2019 is a “business-as-usual” update to the CMI Model. It is based on data for calendar years 1979-2019, rather than 1978-2018 for CMI_2018, but we have not changed the method.

What does CMI_2019 mean for cohort life expectancy?

The positive mortality improvement in 2019 contributes to higher expected mortality improvements in the short to medium term in CMI_2019 than in CMI_2018.

Chart A illustrates the progression of cohort life expectancy for members of defined benefit pension schemes since the first version of the CMI Model, CMI_2009. CMI_2019 produces slightly higher cohort life expectancies than CMI_2018. At age 65, cohort life expectancy is around 1 month higher in CMI_2019 than in CMI_2018 for both males and females.

Cohort life expectancy is a subjective measure that depends on assumed future changes in mortality. Although Chart A shows that cohort life expectancy has typically been falling in recent years, this does not necessarily mean that mortality rates were increasing – instead it typically reflects lower projected rates of future mortality improvement.

Chart A: Cohort life expectancies as at 1 January 2020 at age 65 from CMI_2019 and earlier versions

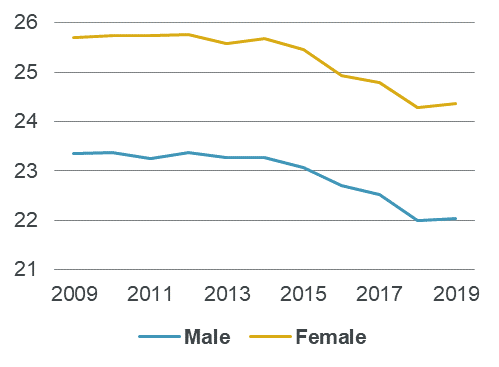
How are these cohort life expectancy figures calculated?

In order to calculate cohort life expectancies, the CMI Model requires its users to make assumptions about current mortality rates and the long-term rate of mortality improvements:

• We have used an illustrative long-term rate of 1.5% a year, as this is a commonly-used value, but the CMI does not comment on its appropriateness or make a recommendation. 
• We have used the latest pension scheme mortality tables, S3PMA and S3PFA. These lead to higher life expectancy than mortality tables for the general population.

2. Historical mortality in England & Wales

How has mortality changed in the past?

Chart B shows standardised mortality rates (SMRs) in the general population of England & Wales from 1979 to 2019, corresponding to the data used to calibrate CMI_2019. Standardised mortality rates have broadly halved over that period. However, the fall in mortality rates has been modest since 2011.

Chart B: Standardised mortality rates since 1979

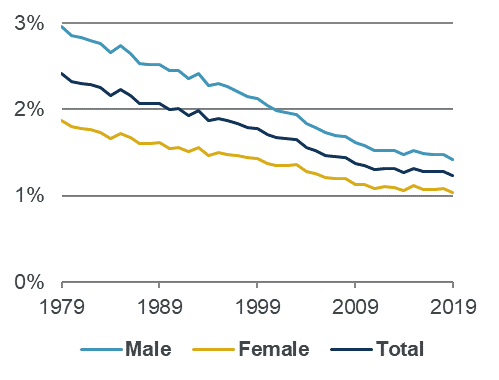
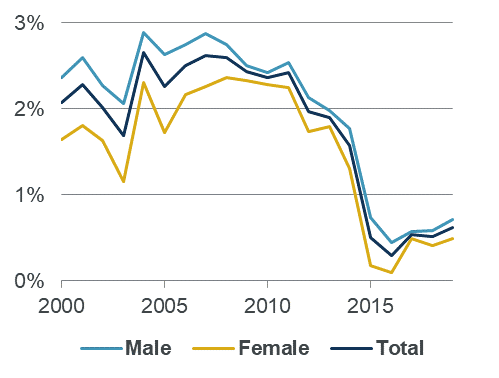
Chart C shows mortality improvements, based on SMRs, for recent five-year periods (e.g. the point for 2019 is the average annual improvement between 2014 and 2019). Mortality improvements for individual years can be very volatile because of short-term effects such as flu and cold winters, so we use five-year averages to reduce the impact of these short-term variations and show the trend more clearly. Average mortality improvements were above 2% a year for most of 2000-2011, but have since fallen to around 0.5% a year.

Chart C: Standardised mortality improvements since 2000

How do mortality improvements vary between different groups of people?

Analysis of data published by the Office for National Statistics (ONS) shows that people aged 65-89 living in less-deprived areas of England & Wales have higher life expectancy than those in more-deprived areas, and since 2001 have experienced mortality improvements about 1% a year higher on average.

Pension scheme members and insurance company policyholders tend to be from less deprived areas on average, so may be expected to experience higher mortality improvements than the general population.

Why have mortality improvements fallen since 2011?

There has been considerable debate about the reasons for lower mortality improvements since 2011.

Most actuaries expected some slowdown in mortality improvements as some of the factors that led to the previous high improvements could not persist. In particular, a large part of the high mortality improvements in the decades before 2011 came from reductions in deaths from circulatory diseases, such as heart attack and stroke. As the proportion of deaths from circulatory diseases fell, subsequent reductions could not contribute as much to overall mortality improvements. A higher proportion of deaths are now caused by cancer and dementia, which have shown lower mortality improvements.

However, the sudden change around 2011 suggests that other factors were involved. For example, economic conditions are likely to have played a part, with GDP falling by over 6% between Q1 2008 and Q1 2009 and subsequently showing lower growth than in previous decades. This has contributed to lower growth in government spending on health and social care than in the preceding period, which may have affected mortality rates.  

3. How the CMI Mortality Projections Model works

Why does the CMI produce the CMI Model?

Actuaries need to make assumptions about mortality rates to help manage pension schemes and insurance companies. They typically do this in two stages – first assessing current mortality rates, and then considering how these rates may change over time. The CMI Model relates only to the second stage.

Many UK pension schemes and insurance companies use the CMI Model either to set their assumptions of how mortality rates will change in future or as a “common language” to communicate assumptions that have been set by another method.

How does the CMI Model work?

The CMI Model enables actuaries to project mortality improvements. They can then apply these improvements to any mortality table to get future mortality rates.

The CMI Model makes its projections by blending between short-term and long-term improvements:

• The short-term improvements are based on historical mortality data. The CMI Model smooths the data, to reduce the effect of short-term volatility, and estimates current mortality improvements by age and gender. The CMI encourages users of the CMI Model to consider adjusting the initial improvements to reflect the portfolio that they are applying it to.
• The long-term improvements are set by assumption, rather than being based on data. As the CMI does not make an assumption for long-term mortality improvements, users need to make their own assessment of the long term. So the CMI Model is a framework for mortality assumptions, rather than giving a single answer, as different users will have different opinions of long-term improvements, and use different methods to form their views.

What mortality data does the CMI Model use?

The CMI Model is based on data for the general population of England & Wales, published by the ONS, but we make a number of estimates and adjustments:

• We adjust the population size at ages 85 and above, and at younger ages where we consider the implied mortality rates to be implausible.
• We make our own estimates of the population and the age distribution of deaths for the final year of data, as the official ONS figures are not available when we update the CMI Model.

Why does the CMI Model use ONS data for England & Wales?

We use population data, rather than datasets collected by the CMI, in order to have a large and consistent dataset over a long period of time.

We use data for England & Wales, rather than the UK, because the relevant data becomes available sooner for England & Wales, so we can keep the CMI Model more up-to-date. We regularly look at results using UK data and, to date, have found that they are little different.

Why is the CMI Model updated?

The CMI Model was introduced in 2009 and has been regularly updated since then:

• We update it annually to take account of mortality data for the most recent calendar year.
• We make occasional changes to the way the CMI Model works to reflect evolving thinking on the most appropriate methodologies. For example, in CMI_2018 we introduced the initial addition to mortality improvements, to help users to tailor the CMI Model to their portfolios, and reduced the period smoothing parameter.

4. Mortality terminology

What is a mortality rate?

A mortality rate is the proportion of people that are expected to die in the following year. For example, if 100 of every 1,000 85-year old males die within a year, then the mortality rate is 10%.

Mortality rates vary by factors including age, gender and socio-economic status; in particular, mortality rates tend to be higher at older ages.

What is a mortality table?

A mortality table is a set of mortality rates for each age, for a particular group of people at a point in time. Mortality tables are usually based on historical data over a number of years, to smooth out short-term volatility. For example, the CMI’s “S3” mortality tables are for pension scheme members in 2009 to 2016.

What is a standardised mortality rate?

Because mortality rates are higher at older ages, changes in the age distribution of a population can lead to apparent changes in average mortality rates, even if mortality rates at each age do not change. A standardised mortality rate (SMR) can be thought of an average mortality rate for a group of lives, assuming that it has a standard age profile. This enables us to make a consistent comparison of mortality rates over time and between groups. We use the age profile of the 2013 European Standard Population for ages 20 to 100.

What is a mortality improvement?

A mortality improvement measures the reduction in mortality rates from one year to the next. Actuaries usually refer to “improvements” rather than “changes” because mortality rates have tended to fall over the long term. A positive improvement represents a fall in mortality rates. A negative improvement represents a rise in mortality rates.

For example, if:

• Last year, 100 of every 1,000 85-year old males died within a year (a mortality rate of 10%).
• This year, only 98 of every 1,000 85-year old males die within a year (a mortality rate of 9.8%).

Then this year’s mortality improvement for 85-year old males is +2%, as there are 2% fewer deaths than in the previous year.

What are period and cohort life expectancies?

Life expectancy is a measure of how much longer, on average, a person in a particular group (by age, gender etc) is expected to live. For example, if females aged 65 have a life expectancy of 25 years, then they are expected to live to 90 on average. Individuals within the group could have much shorter or longer lives.

There are two distinct types of life expectancy, which measure different things:

• Period life expectancy takes no account of future mortality improvements, only historical data. This means that it is an objective measure for comparing mortality between populations and over time, but it isn’t a realistic assessment of how long people may actually live.
• Cohort life expectancy does take account of expected future changes in mortality. This makes it useful to actuaries who need to make realistic assumptions about what may happen in the future. Consequently, the CMI tends to focus on cohort life expectancy. However because future changes in mortality are unknown, cohort life expectancy is subjective, and different actuaries will have different views of cohort life expectancy.